Section Modulus Of Circle

A area in 2 mm 2 i moment of inertia in 4 mm 4 g r radius of gyration in mm y distance of axis to extreme fiber in mm section.

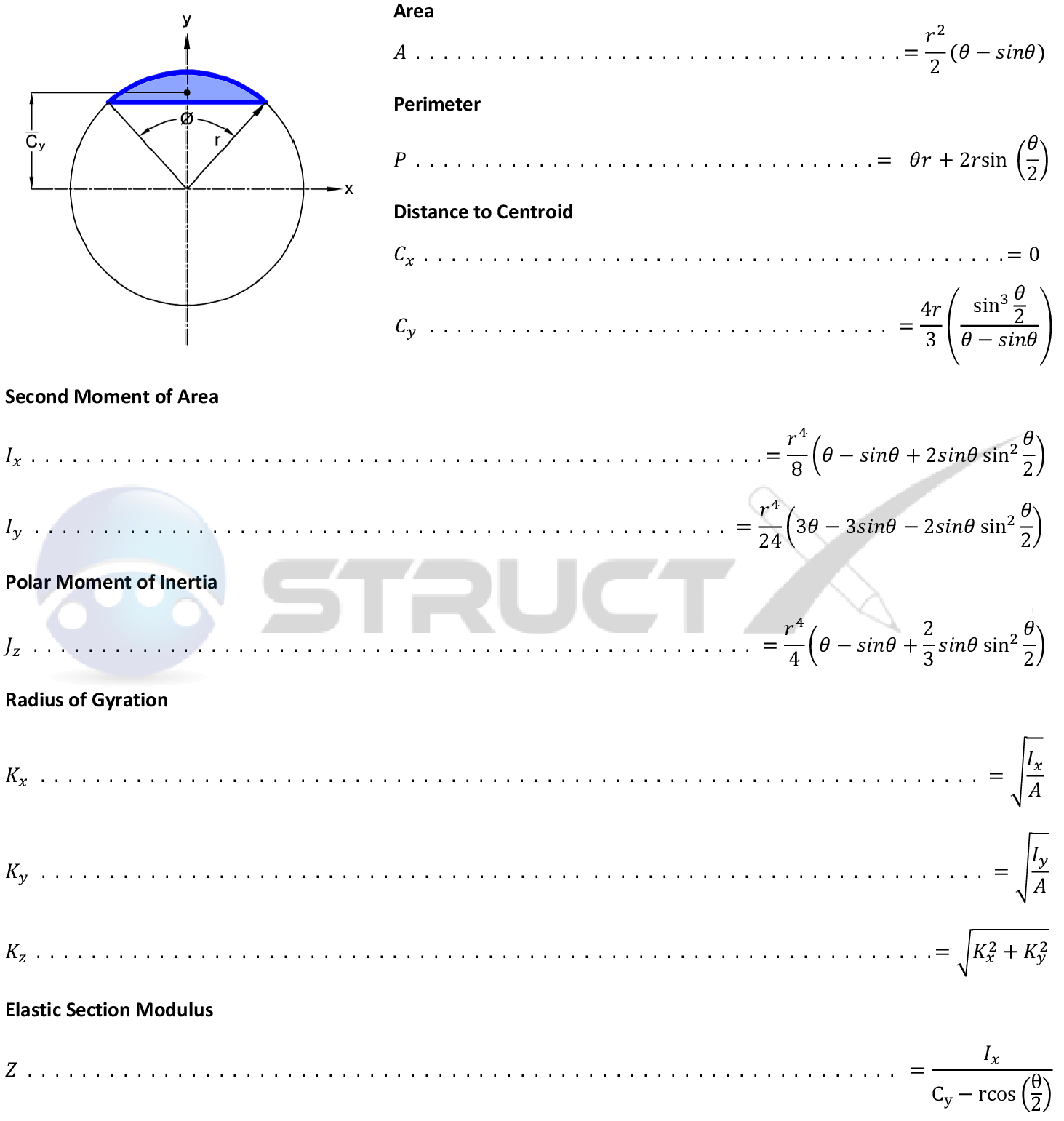
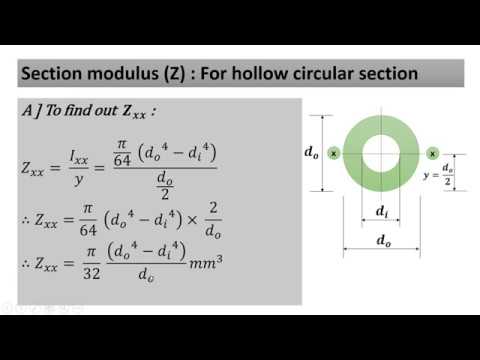
Section modulus of circle. This method may be used to find the approximate value of the polar section modulus of sections that are nearly round. Using the structural engineering calculator located at the top of the page simply click on the the show hide calculator button the following properties can be calculated. Therefore application of the above formula to a circular cross section provides the following expression for the elastic modulus around any centroidal axis.

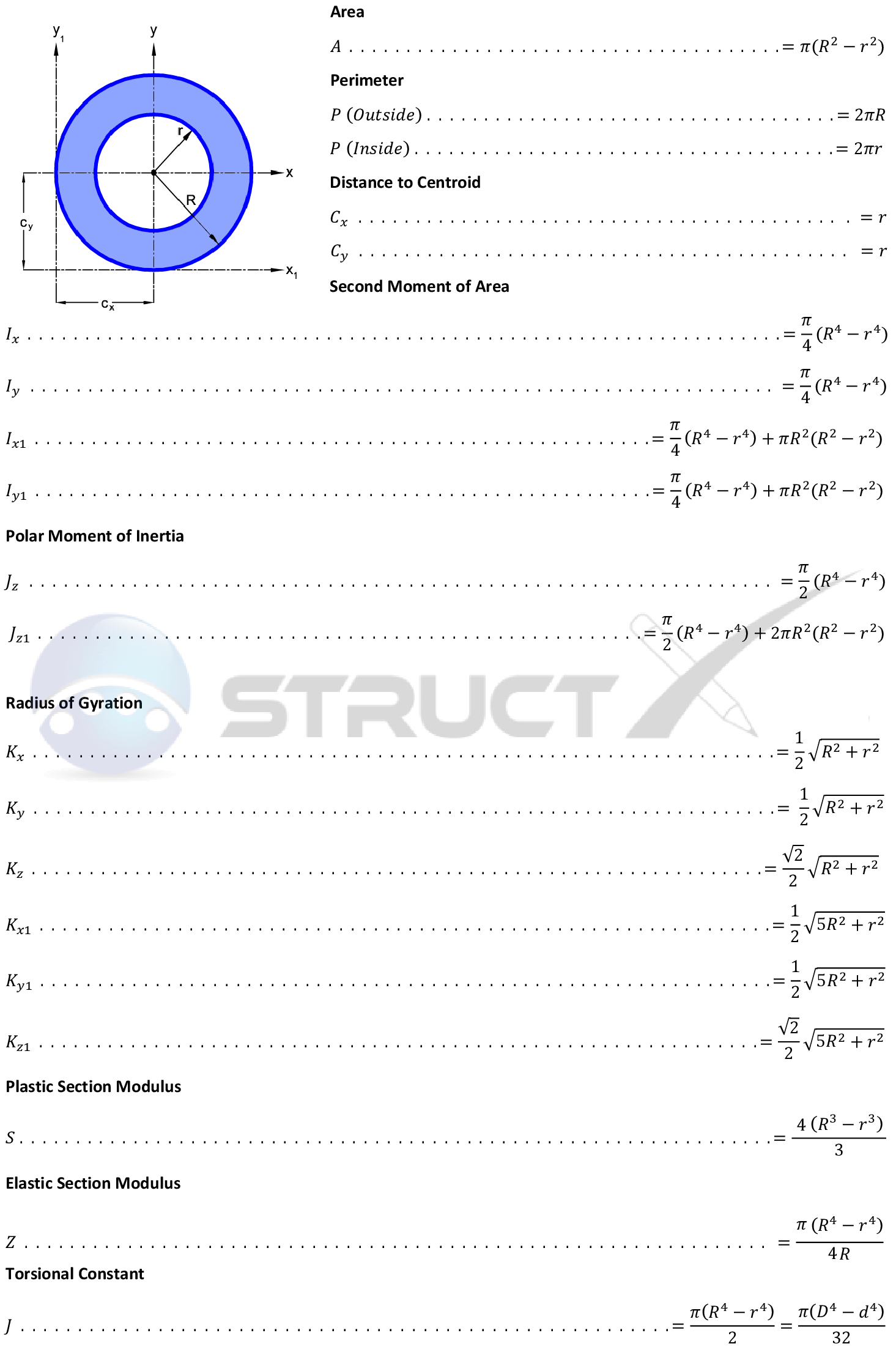
Any relationship between these properties is highly dependent on the shape in question. S plastic section modulus in 3 or mm 3. Online hollow circle annulus property calculator.

There are two types of section moduli the elastic. Z elastic section modulus in 3 or mm 3. Equations for the section moduli of common shapes are given below.

S plastic section modulus in 3 or mm 3. The polar section modulus also called section modulus of torsion z p for circular sections may be found by dividing the polar moment of inertia j by the distance c from the center of gravity to the most remote fiber. Using the structural engineering calculator located at the top of the page simply click on the the show hide calculator button the following properties can be calculated.

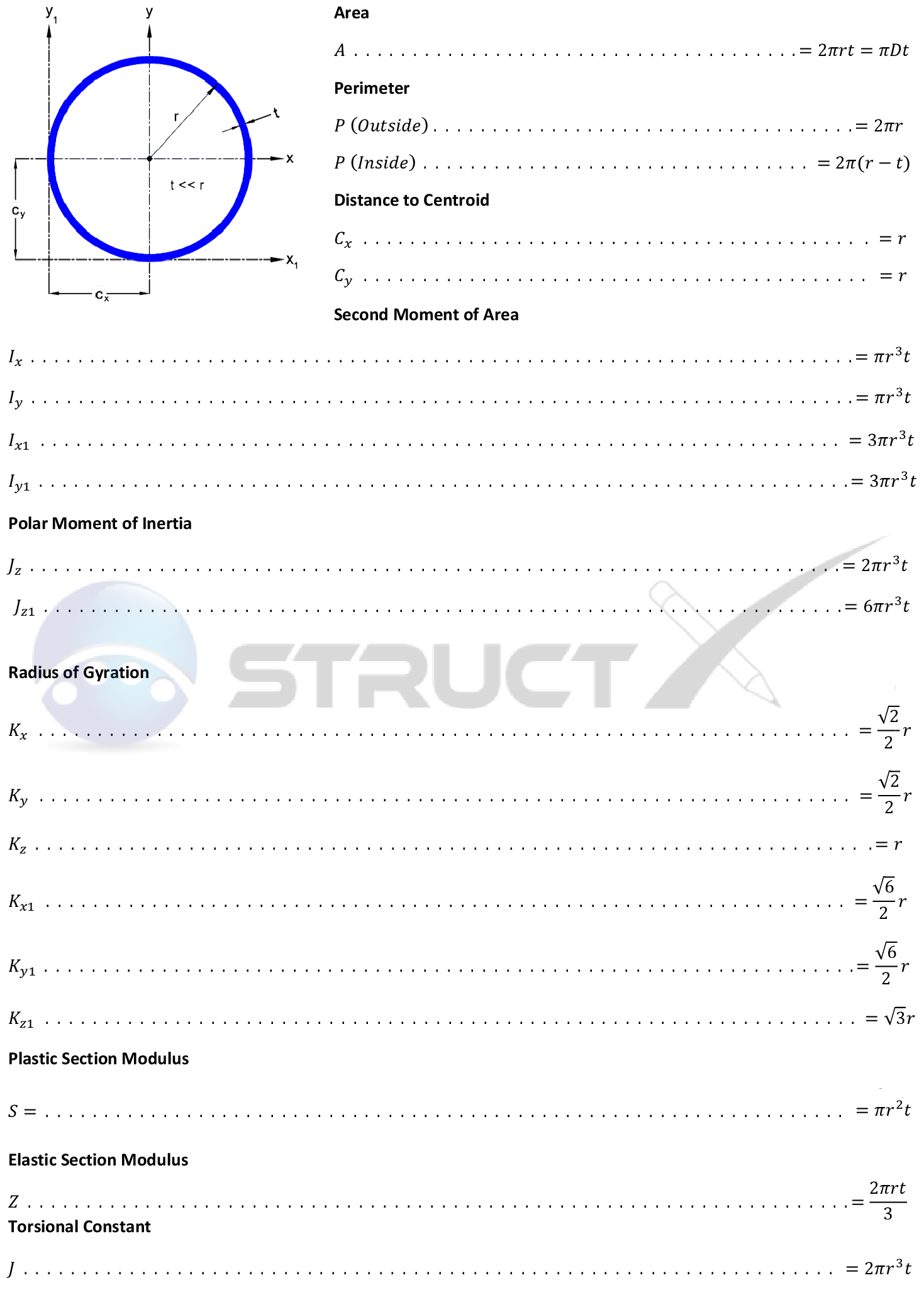
Online circle property calculator. The utility of the section modulus is that it characterizes the bending resistance of a cross section in a single term. Calculate the area of a hollow.

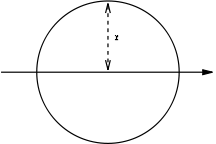
For the circle the bigger distance from center is. Enter the radius of a circle and find its section modulus. Section modulus is a geometric property for a given cross section used in the design of beams or flexural members.